In the interest of not letting too much time go by without a word, a couple of quick updates:
Thesis: Defended
My thesis defense in April went very well. There is a professor in the mathematics department at UNR who has a tendency to ask really persnickety nit-picky questions with annoying regularity (at defenses, colloquia, and informal conversations). He is rarely wrong in his assumptions, but the questions often distract without really adding much to a presentation. I was quite worried that, during the public portion of the defense, he would come up with a humdinger, but it seems that my presentation was well-prepared and clear enough to avoid stepping on any of his pet peeves.
Following the public defense, I was quizzed by my committee for nearly an hour. My impression had always been that this part of the defense was meant to be somewhat adversarial (in essentially the same way that an exam is adversarial). It turns out that we had a very nice conversation about the mathematics presented, my plans for the future, and monkeys.
I was left with a small list of revisions and was then invited to leave the room while my committee came to a decision. Ten minutes later, all of my paperwork was signed, and I was functionally a master of mathematics.
My Defense Slides and Masters Thesis are available.
New Ink
In celebration of completing my masters degree, I got a new tattoo:
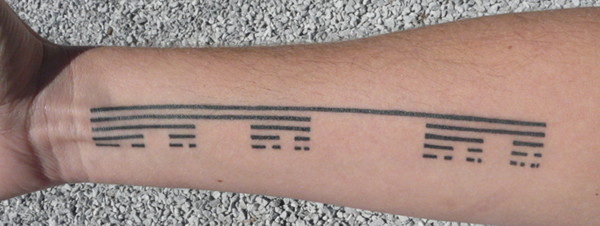
In my thesis research, asymmetric Cantor sets proved to be interesting objects of study. An asymmetric Cantor set can be described as the attractor of an iterated function system consisting of only two maps. Such systems are highly regular (where “regular” is used here in a somewhat informal sense), thus one might hope that they are well-behaved with respect to certain notions of “well-behaved” that are explored in the thesis. As described in the thesis, we have no such luck, which was, to me, a really surprising result.
The tattoo itself is the first five stages in the construction of a particular asymmetric Cantor set (that obtained by leaving the first half and last third of each interval at each stage of construction). This particular set does not exactly behave badly in the sense of the thesis, but it is an aesthetically pleasing member of the family of sets I studied, thus it seems to be an appropriate symbol of the research that I have been working on for the last two years.
