Image Details
Click the image above to obtain a larger version (approx. 9 MB).
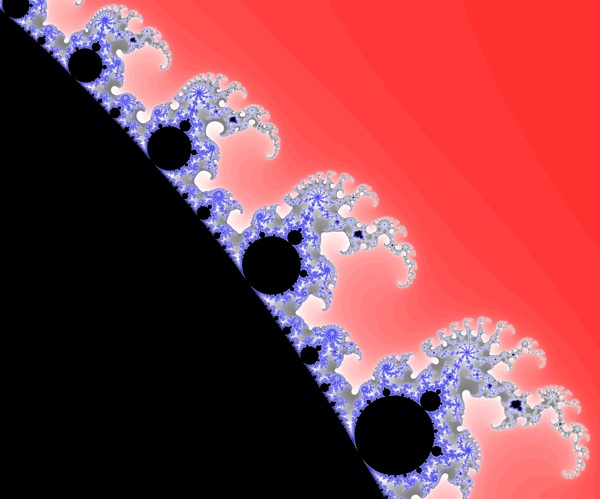
This week’s image is from a section of the Mandelbrot set known as Elephant Valley. Elephant Valley lies near the positive real axis (i.e. the x-axis) in the complex plane, and is so named because the figures along the border of the Mandelbrot set in this region resemble elephants. There are a couple of interesting features that are worth noting. First, each elephant seems to be standing on a black ball. These balls all look about the same, which demonstrates one of the defining features of a fractal, i.e. self-similarity.
Second, each of the elephants look about the same, but there is a difference: count the prominences on the back of each elephant. The elephant on the far right has 8 prominences (including the head), while the last complete elephant on the left has 11 prominences. Superficially, the elephants all look identical, but there are minor and predictable variations. If we were to see more of the Mandelbrot set to the left, and zoom in more, we could find an infinite number of elephants, and each elephant would have one more prominence than the elephant directly to its right.
Third, if you examine the larger version of the image, you might note that each of the prominences noted above is, in fact, a smaller copy of the elephant that it is standing on (more or less). For instance, each prominence on the back of the far right elephant is, in fact, a smaller elephant with 8 prominences on each of its back. And, in turn, each of those prominences is another miniature elephants. With enough computing power, we could continue to find more and more elephants on the backs of elephants on the backs of elephants. It’s turt—er… elephants—all the way down!
Finally, once again examine the larger image. Look at the balls that the elephants are standing on. There are more elephants! Moreover, these little elephants have much the same property as the ones discussed above—they are made up of elephants upon elephants. Once again, we can see the fractal self-similarity.
Cool, no?